
Negative times Negative: How should we compute \(\left(-2\right) \times \left(-3\right)\)?Īpplying the commutative law and thinking of this as \(\left(-3\right) \times \left(-2\right)\) does not help in this case. Negative times Positive: This is problematic: \(\left(-2\right)\times 3=?\)īut it does seem compelling to say that the commutative law, \(a \times b = b\times a\), should hold for all types of numbers, including negative numbers. Most people agree we should stay with this idea.

Positive times Negative: It does seem compelling to hold on to the “repeated addition” notion for the product of a negative and a positive: To approach this we first have to be clear on what features of arithmetic we feel should still be true. Asking “What does the multiplication of negative numbers mean?” is a misleading question and it is not the question to ask at this point of our work: we’re still just trying to grapple with the issue of what multiplication is in the world of negative numbers could be. How would we like multiplication to behave?Ĭomment: Let me stress this point. So we have to engage in a sophisticated shift of thinking, letting go of the question What is multiplication? to ask instead: The truth is that multiplication has no meaning here in context of repeated addition. We have entered new territory and if we want to open up our world to new types of numbers it is not surprising that previously concrete, literal definitions begin to flail. What could \(\left(-2\right) \times 3\) mean? “Negative two groups of three” makes no sense.Īnd \(\left(-2\right) \times \left(-3\right)\) is equally strange: “negative two groups of negative three.” Interpreting negative times a positive and negative times negative through repeated addition, however, is problematic.
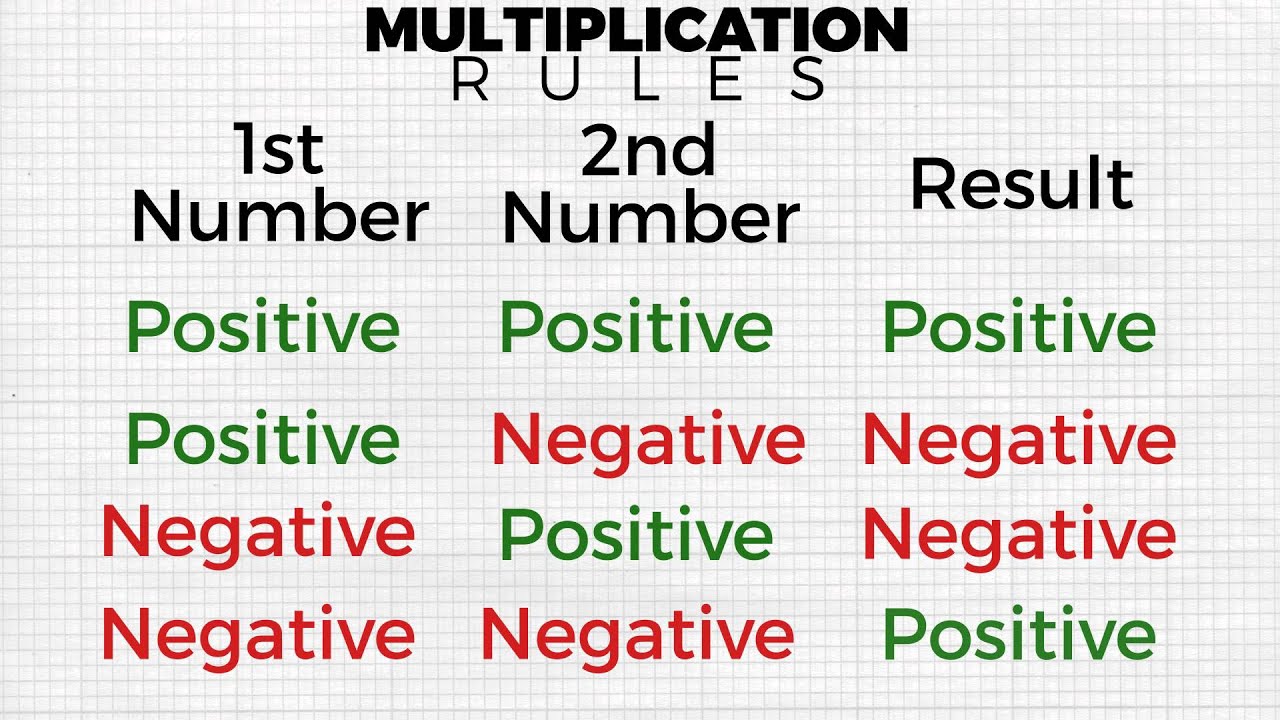
For example, \(2 \times \left(-3\right)\) can be read as “two groups of negative three” and so is computed as \(2 \times \left(-3\right)=-3+\ -3=-6\). Using piles and holes this looks like: Repeated addition allows us to multiply a positive number and a negative number. Question: How would you convince a young student that 193 groups of 307 is sure to have the same value as 307 groups of 193? Why do we wish that believe that \(a \times b = b \times a\) for counting numbers? (HINT: Put dots in rectangular arrays.) The computation is quite different with the numbers 5 and 4 serving switched roles. It is actually quite a surprise that \(5 \times 4\), “five groups of four,” gives the same numerical answer as four groups of five. In the early curriculum multiplication is introduced in the context of whole counting numbers and is appropriately defined there as repeated addition. For instance, \(4 \times 5\) is read as “four groups of five” and is computed as such: \(4 \times 5 = 5 + 5 + 5 + 5 = 20\). MULTIPLYING POSITIVE AND NEGATIVE NUMBERS That is, we like to believe that basic laws such as \(a\times b=b\times a\) and \(a \times 1=a\) and \(a \times 0=0\) hold for all numbers, both positive and negative, and that we can expand brackets even with negative entries, and so on. Of course, these rules assume we know a priori know how to multiply with negative numbers. When we discover negative numbers we naturally, without question even, assume they obey the same laws of arithmetic as the ordinary positive counting numbers.


 0 kommentar(er)
0 kommentar(er)
